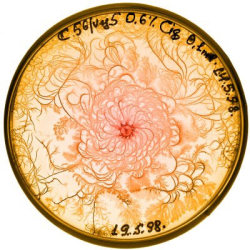
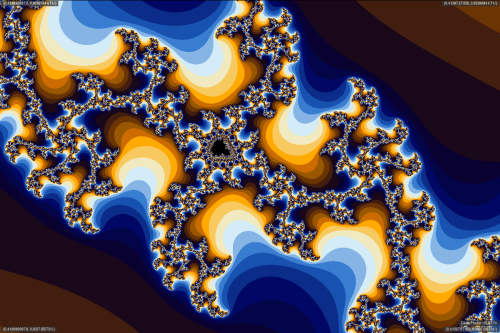
So What Is a Fractal, Anyway?

So What Is a Fractal, Anyway?

It is my firm conviction that mathematics underpins everything in the universe. Mathematics was intrinsically designed to explain the world; Newton invented differential and integral calculus, for example, to explain why the orbits of the planets were in the shape of an ellipse. Understanding the mathematics behind phenomena will not make them any less beautiful; it will make them more beautiful in that the complexity of our world is better understood and appreciated.

To put it simply, fractals are self-similar and have an unusual relationship with space - kind of like the ‘It’s Complicated’ relationship status on Facebook. Self-similar, in this case, means that fractals have something called irreducible complexity: No matter how far you “zoom in” to a fractal pattern, the structure will be exactly or approximately similar to the fractal itself. In order to understand a fractal’s unusual relationship with space, however, we’re going to need to consider the concept ofdimensions.

Dimensions, in their simplicity, are a very interesting mathematical concept. What, for example, makes a line one-dimensional, and a plane two-dimensional? Note that lines and planes are both self-similar, as outlined by the definition above. The differences actually lie in their self-similarity; a line can be broken down into N self-similar pieces, each with a magnification factor of N; a plane can be broken down into N^2 similar pieces, each with a magnification factor N; and a cube into N^3 pieces, with the same magnification factor N. A magnification factor will be defined here as the amount you have to “zoom in” to regain the original structure.

Therefore, dimensions of self-similar objects are simply the exponent of the number of self-similar pieces with magnification factor N into which the figure may be broken. So the line segment mentioned before exists in one dimension; the plane in two dimensions; and the cube in three dimensions. Not too bad, right?

Well, here’s the complicated part. Fractals can exist in non-integer dimensions.

For example, we can consider the Sierpinski Triangle, which looks like this:

If we accept our original ideas about dimensions to be valid, then thedimension of the Sierpinski Triangle - which, by our definition, exhibits a fractal pattern - would be governed by:

Fractals have an “unusual relationship with space” because they - as shown by the Sierpinski Triangle - can exist in non-integer dimensions. Amazing, right?

The three bottom images above are taken from the Mandelbrot Set - which has a topological dimension of 2 (meaning that it’s visualised, and pictured, in two dimensions) but has a fractal dimension that’s much more complex. Fractals from the Mandelbrot set - and other fractal patterns - are found throughout nature, including in the growth patterns of bacteria at the top of this post! Other examples include unfurling ferns, and the inside of certain types of seashells. It’s even been shown that the small motions of our eyes follow a fractal pattern - which is why we find things that exist in a fractal pattern aesthetically pleasing.

Next time you see a fractal pattern, remember that it doesn’t just exist topologically as you see it - it’s fractal dimension could be 1.58, or 1.7!The maths behind fractal images are just as beautiful as the images themselves. In fact, in my opinion they enhance the beauty of the images my eye naturally finds aesthetically pleasing.

Images: The top two images shown are of bacteria cultures exhibiting fractal growth due to environmental stress. The bottom three images are from the Mandelbrot Set.